11.14. Piece-wise Functions
A piece-wise function is defined as a sequence of intervals and each interval is a subset of the domain of the function. Some examples of piece-wise functions are:
Sketching a piece-wise function means sketching the graphs of several functions with applied domains. Use the following steps in Graph mode:
1) Enter the equation for the first interval.
The default domain is from -∞ to +∞.
Change the domain to the equation's given interval.
Tap the down-arrow on the left of the input field to be able to change the equation's domain.
2) Enter the equation for the second interval.
Change the default domain based on the given second interval.
3) Use the same procedure for each of the following intervals.
Examples
Sketch the graph of each piece-wise function below.
1) Enter the equation for the first interval.
The default domain is from -∞ to +∞.
Change the domain to the equation's given interval.
Tap the down-arrow on the left of the input field to be able to change the equation's domain.
2) Enter the equation for the second interval.
Change the default domain based on the given second interval.
3) Use the same procedure for each of the following intervals.
Examples
Sketch the graph of each piece-wise function below.
Solutions
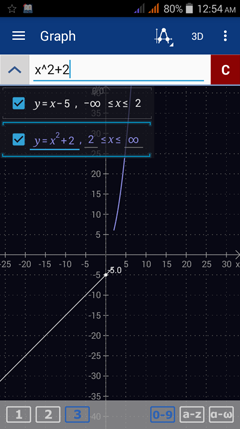
1) Type x - 5.
Tap the down-arrow on the left of the input field.
Keep -∞ as the lower bound but change the upper bound from ∞ to 2.
Hit the enter key three times.
Type x^2 + 2.
Tap the down-arrow on the left of the input field.
Change the lower bound from -∞ to 2 and keep the upper bound at ∞.
Hit the enter key three times.
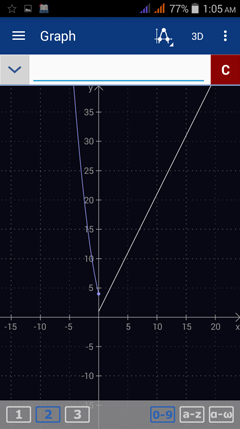
2) Enter |2x + 1| by using the absolute value key |x|. It should appear as abs(2x + 1).
Tap the down-arrow on the left of the input field.
Change the lower bound from -∞ to 0 and keep the upper bound at ∞.
Hit the enter key three times.
Enter (x - 2) ^ 2.
Tap the down-arrow on the left of the input field.
Keep the lower bound at -∞ but change the upper bound from ∞ to 0.
Hit the enter key three times.
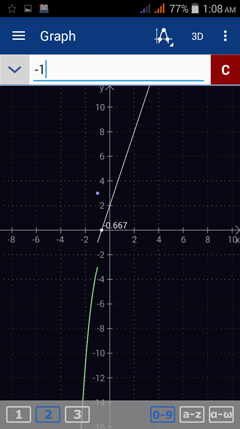
3) Enter 3x + 2.
Tap the down-arrow on the left of the input field.
Change the lower bound from -∞ to -1 and keep the upper bound at ∞.
Hit the enter key three times.
Plot the point (-1, 3) by typing [-1, 3].
Hit the enter key.
Enter x^3 + x - 1.
Tap the down-arrow on the left of the input field.
Keep the lower bound at -∞ and change the upper bound from ∞ to -1.
Hit the enter key three times.