6.2 Fractions
6.2.1 Mixed Fractions
6.2.2 Complex Fractions
A fraction is used to represent parts of a whole. If one out of four slices of a circle is shaded, the shaded part is 1/4 of the circle. The 1 is the numerator and the 4 is the denominator. More generally, fractions are written in the form a/b.
Fractions can be proper, improper or mixed. A proper fraction is a fraction with a numerator lower than its denominator such as 1/4 in the example above. An improper fraction is a fraction with a numerator lower than its denominator such as 3/2. A mixed fraction contains a whole number and a proper fraction. The improper fraction 3/2 would be written 1 1/2 as a mixed fraction.
Enter a proper or improper fraction in the form a/b by using the fraction bar (/). If the numerator or denominator is expressed as a series of operations, enclose the arguments in parentheses.
Example
Perform the following operations. Express the final answer in its simplest form.
Solution
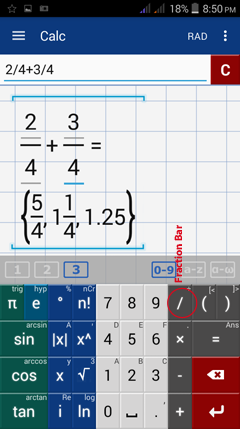
1) Enter the expression as it appears in the problem: 2/4 + 3/4
1) Enter the expression as it appears in the problem: 2/4 + 3/4
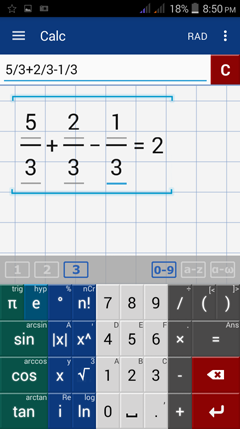
2) Enter the expression as it appears in the problem: 5/3 + 2/3 - 1/3
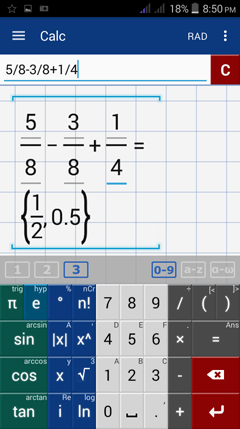
3) Enter the expression as it appears in the problem: 5/8 - 3/8 + 1/4
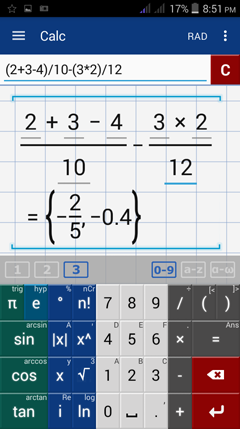
4) Enter the expression as it appears in the problem: (2 + 3 - 4)/10 - (3 * 2)/12
Multiplying Fractions
To multiply fractions by hand:
1) Convert all mixed numbers to improper fractions.
2) Multiply the numerators across.
3) Multiply the denominators across.
4) Simplify the result if necessary.
In general: a/b × c/d = ac/bd
To multiply fractions in this app:
1) Enter the fractions as they appear in the problem.
2) Use the fraction bar ( / ) to separate the numerator from the denominator.
3) Use the multiplication symbol ( × ) to multiply.
4) The answer will be simplified automatically.
Examples
To multiply fractions by hand:
1) Convert all mixed numbers to improper fractions.
2) Multiply the numerators across.
3) Multiply the denominators across.
4) Simplify the result if necessary.
In general: a/b × c/d = ac/bd
To multiply fractions in this app:
1) Enter the fractions as they appear in the problem.
2) Use the fraction bar ( / ) to separate the numerator from the denominator.
3) Use the multiplication symbol ( × ) to multiply.
4) The answer will be simplified automatically.
Examples
Solution
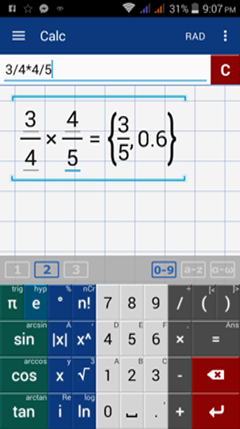
Enter the expression as it appears in the problem: 3/4 * 4/5
Enter the expression as it appears in the problem: 3/4 * 4/5
Solution
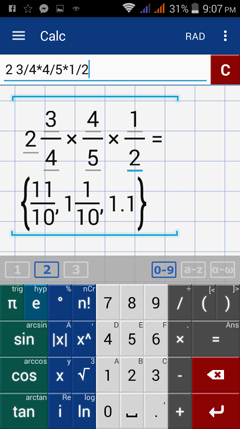
Enter the expression as it appears in the problem: 2 3/4 * 4/5 * 1/2 with a space between the whole number and fraction in the mixed number.
Enter the expression as it appears in the problem: 2 3/4 * 4/5 * 1/2 with a space between the whole number and fraction in the mixed number.
Solution
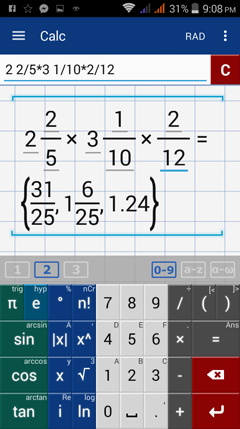
Enter the expression as it appears in the problem: 2 2/5 * 3 1/10 * 2/12
Enter the expression as it appears in the problem: 2 2/5 * 3 1/10 * 2/12
Solution
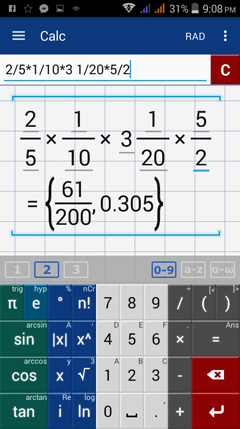
Enter the expression as it appears in the problem: 2/5 * 1/10 * 3 1/20 * 5/2
Enter the expression as it appears in the problem: 2/5 * 1/10 * 3 1/20 * 5/2
Solution
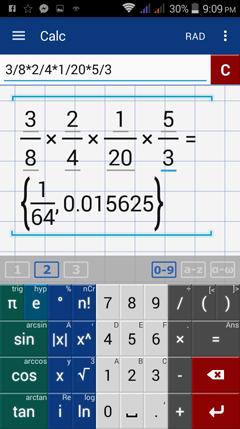
Enter the expression as it appears in the problem: 3/8 * 2/3 * 1/20 * 5/3
Enter the expression as it appears in the problem: 3/8 * 2/3 * 1/20 * 5/3
Dividing Fractions
To divide fractions by hand:
1) Convert all mixed numbers to improper fractions.
2) Take the reciprocal of the divisor (the fraction after the division symbol).
3) Change the operation from division to multiplication.
4) Follow multiplication rule.
5) Simplify the result if necessary.
In general: a/b ÷ c/d = a/b × d/c = ad/bc.
To divide fractions in this app:
1) Enter the fractions as they appear in the problem.
2) Use the fraction bar ( / ) to separate the numerator from the denominator.
3) Use the division symbol ( ÷ ), the second function of the fraction bar.
4) The answer will be simplified automatically.
Examples
To divide fractions by hand:
1) Convert all mixed numbers to improper fractions.
2) Take the reciprocal of the divisor (the fraction after the division symbol).
3) Change the operation from division to multiplication.
4) Follow multiplication rule.
5) Simplify the result if necessary.
In general: a/b ÷ c/d = a/b × d/c = ad/bc.
To divide fractions in this app:
1) Enter the fractions as they appear in the problem.
2) Use the fraction bar ( / ) to separate the numerator from the denominator.
3) Use the division symbol ( ÷ ), the second function of the fraction bar.
4) The answer will be simplified automatically.
Examples
Solution
Enter the expression as it appears in the problem: 3/8 ÷ 2/4
Enter the expression as it appears in the problem: 3/8 ÷ 2/4
Solution
Enter the expression as it appears in the problem: 2 3/5 ÷ 2/8. Type the division sign by tapping the fraction bar ( / ) twice.
Enter the expression as it appears in the problem: 2 3/5 ÷ 2/8. Type the division sign by tapping the fraction bar ( / ) twice.
Solution
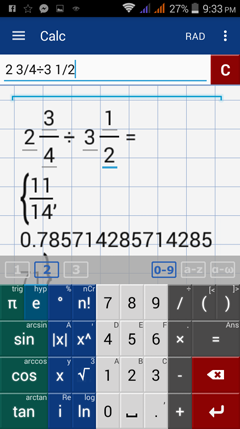
Enter the expression as it appears in the problem: 2 3/4 ÷ 3 1/2
Enter the expression as it appears in the problem: 2 3/4 ÷ 3 1/2
Solution
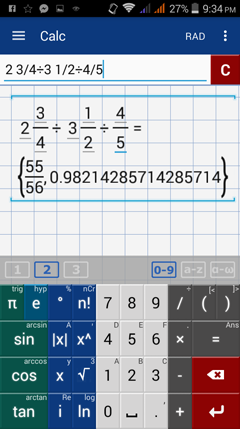
Enter the expression as it appears in the problem: 2 3/4 ÷ 3 1/2 ÷ 4/5
Enter the expression as it appears in the problem: 2 3/4 ÷ 3 1/2 ÷ 4/5
Solution
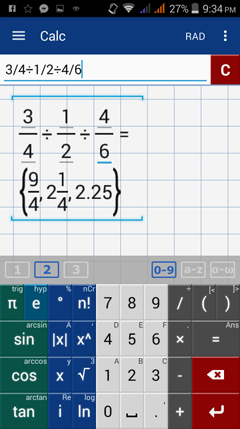
Enter the expression as it appears in the problem: 3/4 ÷ 1/2 ÷ 4/6
Enter the expression as it appears in the problem: 3/4 ÷ 1/2 ÷ 4/6