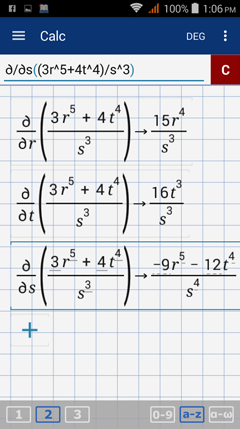17.6. Total Derivates - Graphing Calculator by Mathlab: User Manual

17.6. Total Derivatives

If a function has multiple independent variables, the total derivative is the sum of the partial derivatives with respect to each variable.

Illustrative Example
Find the total derivative of the given function.

$f(x,y)=3x^4+4x^2y^6$

Manual solution

1) Find the partial derivatives with respect to x and y.
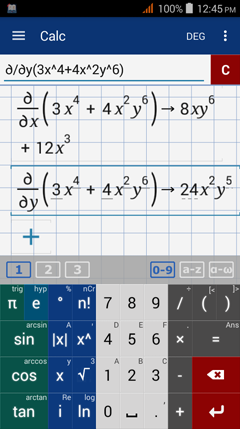
Partial derivative with respect to x, Δx = (12x^3 + 8xy^6) dx
Partial derivative with respect to y, Δy = 24x^2y^5 dy
2) Add the two partial derivatives: (12x^3 + 8xy^6) dx + 24x^2y^5 dy

Note: The calculator doesn't display dx and dy.

Calculator solution

Partial derivative with respect to x

Partial derivative with respect to y

Total derivative

$\partial /\partial x\left ( 3x^4+4x^2y^6 \right )$

$\partial /\partial y\left ( 3x^4+4x^2y^6 \right )$

$\left ( 12x^3+8xy^6 \right )\, dx+24x^2y^5\, dy$

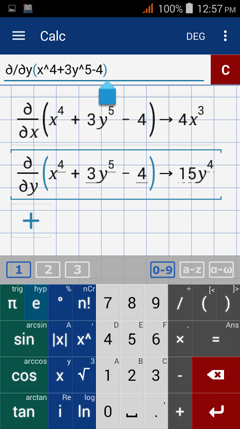
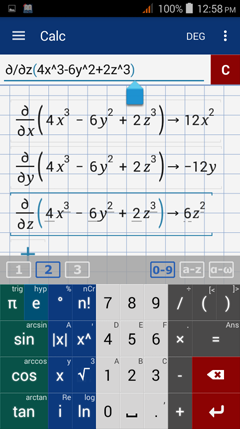
More Examples
Find the total derivative of each function below.

$1)f(x,y)=x^4+3y^5-4$

Calculator Syntax:

$\partial /\partial x\left (x^4+3y^5-4 \right )$

$\partial /\partial y\left (x^4+3y^5-4 \right )$

Total derivative

$4x^3\: dx+15y^4\: dy$

$2) f(x,y,z)=4x^3-6y^2+2z^3$

Calculator Syntax:

Total derivative

$\partial /\partial x\left (4x^3-6y^2+2z^3 \right )$

$\partial /\partial y\left (4x^3-6y^2+2z^3 \right )$

$\partial /\partial z\left (4x^3-6y^2+2z^3 \right )$

$12x^2\: dx-12y\, dy+6z^2\: dz$

$3) f(r,s,t)=\frac{3r^5+4t^4}{s^3}$

Calculator Syntax:

Total derivative

$\partial /\partial r\left (\frac{3r^5+4t^4}{s^3} \right )$

$\partial /\partial s\left (\frac{3r^5+4t^4}{s^3} \right )$

$\partial /\partial t\left (\frac{3r^5+4t^4}{s^3} \right )$

$\frac{15r^4}{s^3}\: dr+\frac{16t^3}{s^3}\: dt-\frac{9r^5+12t^4}{s^4}\: ds$

next: 18. definite integrals >

list of contents