15.7. Limit of an Absolute Value Function
The absolute value of a number is the distance of the number from zero, meaning that the absolute values of 6 and -6 are 6.
An absolute value function is defined as:
An absolute value function is defined as:
Limit of an Absolute Value Function as x Approaches a Real Number c
Because the argument of an absolute value function may be positive or negative, we have to satisfy both cases: when x > 0 and x < 0.
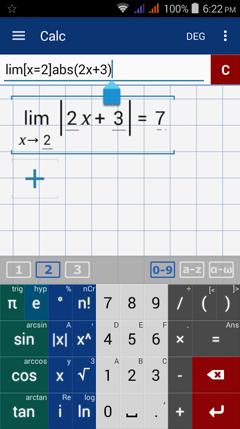
Suppose we want to find the limit of |2x + 3| as x approaches 2 as follows:
Because the argument of an absolute value function may be positive or negative, we have to satisfy both cases: when x > 0 and x < 0.
Suppose we want to find the limit of |2x + 3| as x approaches 2 as follows:
To calculate the limit, we have to separate the absolute value into two cases:
Case 1: 2x + 3 if 2x + 3 > 0
Case 2: -(2x + 3) if 2x + 3 < 0
Case 1: 2x + 3 if 2x + 3 > 0
Case 2: -(2x + 3) if 2x + 3 < 0
Because we are looking for the limit as x approaches 2, it falls into the first case since 2 > -3/2. Therefore to find the limit, we have to plug 2 using |2x + 3|. The limit is |2*2 + 3| = 7.
More Examples
Find the limit of each absolute value function if it exists.
Find the limit of each absolute value function if it exists.
Calculator solution
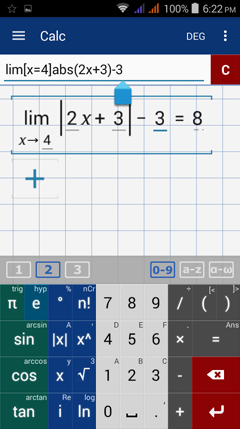
Type in: lim [ x = 4 ] abs( 2x + 3 ) - 3
Case 1: 2x + 3 if 2x + 3 > 0 or x > -3/2
Case 2: -(2x + 3) if 2x + 3 < 0 or x < -3/2
Since 4 > - 3/2, we evaluate the expression 2x + 3 at x = 4.
|2(4) + 3| - 3 = 11 - 3 = 8.
Type in: lim [ x = 4 ] abs( 2x + 3 ) - 3
Case 1: 2x + 3 if 2x + 3 > 0 or x > -3/2
Case 2: -(2x + 3) if 2x + 3 < 0 or x < -3/2
Since 4 > - 3/2, we evaluate the expression 2x + 3 at x = 4.
|2(4) + 3| - 3 = 11 - 3 = 8.
Calculator solution
Type in: lim [ x = -3 ] ( ( abs( x + 1 ) + 3 / x )
Case 1: x + 1 if x > - 1
Case 2: -(x + 1) if x < -1
Since -3 < - 1, we evaluate -(x + 1) at x = -3.
-(-3 + 1) + 3/(-3) = -(-2) - 1 = 2 - 1 = 1.
Type in: lim [ x = -3 ] ( ( abs( x + 1 ) + 3 / x )
Case 1: x + 1 if x > - 1
Case 2: -(x + 1) if x < -1
Since -3 < - 1, we evaluate -(x + 1) at x = -3.
-(-3 + 1) + 3/(-3) = -(-2) - 1 = 2 - 1 = 1.
Calculator solution
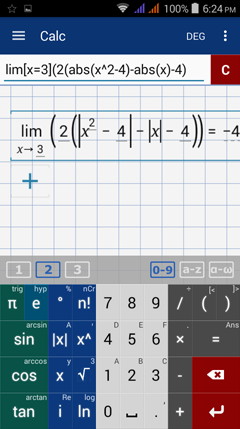
Type in: lim [ x = 3 ] ( 2 ( abs( x^2 - 4 ) - abs( x ) - 4 ) )
Type in: lim [ x = 3 ] ( 2 ( abs( x^2 - 4 ) - abs( x ) - 4 ) )
Calculator solution
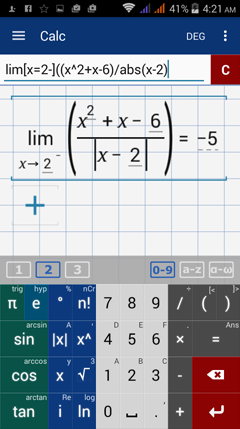
Type in: lim [ x = 2 - ] ( ( x^2 + x - 6 ) / abs( x - 2 ) )
Type in: lim [ x = 2 - ] ( ( x^2 + x - 6 ) / abs( x - 2 ) )