19.5.4. Mode
The mode of a data set is the value that appears most frequency. For example, in the data set: 12, 3, 4, 5, 6, 12, 8, 9, 12 the number 12 appears three times while every other value appears once, so 12 is the mode.
To calculate the mode of a given data set, hold the factorial key (n!) and select mode or type "mode" using the a-z keyboard. Enter the data set in parentheses and separate data points using a comma.
Note: The mode function only works for unimodal data sets i.e. data sets that have one mode.
Illustration
Calculate the mode of the following data set.
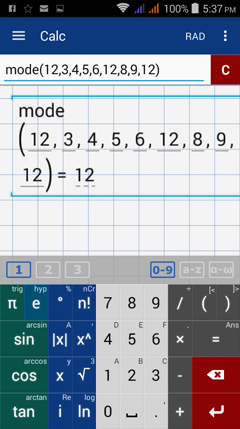
12, 3, 4, 5, 6, 12, 8, 9, 12
Solution
Select mode under the factorial key (n!) or type "mode" using the a-z keyboard.
Then type the following: 12, 3, 4, 5, 6, 12, 8, 9, 12
To calculate the mode of a given data set, hold the factorial key (n!) and select mode or type "mode" using the a-z keyboard. Enter the data set in parentheses and separate data points using a comma.
Note: The mode function only works for unimodal data sets i.e. data sets that have one mode.
Illustration
Calculate the mode of the following data set.
12, 3, 4, 5, 6, 12, 8, 9, 12
Solution
Select mode under the factorial key (n!) or type "mode" using the a-z keyboard.
Then type the following: 12, 3, 4, 5, 6, 12, 8, 9, 12
Examples
Find the mode of each data set.
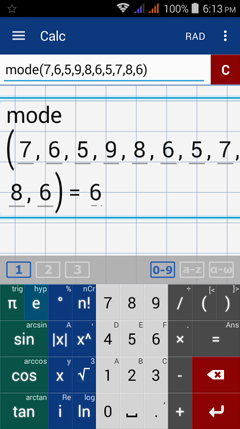
1) The shoe sizes of ten people in a survey are: 7, 6, 5, 9, 8, 6, 5, 7, 8, 6.
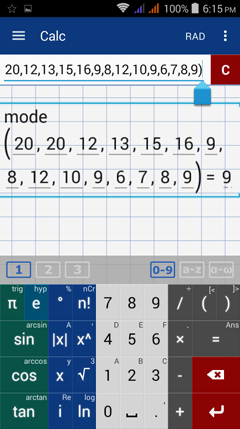
2) The math test scores (out of 20) of 15 students are: 20, 20, 12, 13, 15, 16, 9, 8, 12, 10, 9, 6, 7, 8, 9.
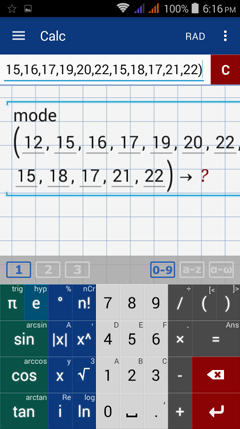
3) The ages of 12 students at a gathering are: 12, 15, 16, 17, 19, 20, 22, 15, 18, 17, 21, 22.
Calculator solutions
1) Hold the factorial key (n!) and select mode.
Then enter the data set: 7, 6, 5, 9, 8, 6, 5, 7, 8, 6
Find the mode of each data set.
1) The shoe sizes of ten people in a survey are: 7, 6, 5, 9, 8, 6, 5, 7, 8, 6.
2) The math test scores (out of 20) of 15 students are: 20, 20, 12, 13, 15, 16, 9, 8, 12, 10, 9, 6, 7, 8, 9.
3) The ages of 12 students at a gathering are: 12, 15, 16, 17, 19, 20, 22, 15, 18, 17, 21, 22.
Calculator solutions
1) Hold the factorial key (n!) and select mode.
Then enter the data set: 7, 6, 5, 9, 8, 6, 5, 7, 8, 6
2) Hold the factorial key (n!) and select mode.
Then enter the data set: 20, 20, 12, 13, 15, 16, 9, 8, 12, 10, 9, 6, 7, 8, 9
3) Hold the factorial key (n!) and select mode.
Then enter the data set: 12, 15, 16, 17, 19, 20, 22, 15, 18, 17, 21, 22
Since the data set has three modes (15, 17, and 22), the app can't display a result.