16.3. Third and Higher Derivatives
To find:
1) First derivative - tap the exponent key (x^) twice
2) Second derivative - tap the exponent key (x^) three times
3) Third derivative - take the second derivative and then the first derivative of the second derivative.
tap x^ three times, then tap x^ twice
4) Fourth derivative - take the second derivative and then the second derivative of the second derivative.
tap x^ three times, then tap x^ three times again
Examples
Find the following derivatives.
1) First derivative - tap the exponent key (x^) twice
2) Second derivative - tap the exponent key (x^) three times
3) Third derivative - take the second derivative and then the first derivative of the second derivative.
tap x^ three times, then tap x^ twice
4) Fourth derivative - take the second derivative and then the second derivative of the second derivative.
tap x^ three times, then tap x^ three times again
Examples
Find the following derivatives.
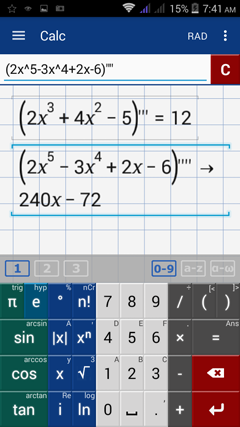
1. f(x) = (2x3 + 4x2 – 5 ). Find f '''(x)
2. f(x) = (2x5 – 3x4 + 2x – 6). Find f 4(x)
Calculator solutions
Enter each expression as given with one per line. Enter the expression in parentheses. Type a third derivative by tapping the exponent key (x^n) twice and then three times. Type a fourth derivative by tapping the exponent key (x^n) three times and then three times again.
1) Type: (2x^3 + 4x^2 - 5)'''
3) Type: (2x^5 - 3x^4 + 2x - 6)''''
Enter each expression as given with one per line. Enter the expression in parentheses. Type a third derivative by tapping the exponent key (x^n) twice and then three times. Type a fourth derivative by tapping the exponent key (x^n) three times and then three times again.
1) Type: (2x^3 + 4x^2 - 5)'''
3) Type: (2x^5 - 3x^4 + 2x - 6)''''